Einstein Hat Awards (entries 159-126)
Thank you to our sponsors, XTX Markets, UK Maths Trust, National Museum of Mathematics (MoMath.org), Amplify, G-Research, Jane Street and Dexter and Deborah Senft.
Contributors - Geoff Smith, Simon Coyle, Samuel Monnier, Dianne Flatt, Cindy Lawrence, Chaim Goodman-Strauss, Guillermo Acevedo, Kit Reagan, Hayley Richardson, Philipp Legner, Craig Kaplan, Robert Fathauer, Yoshiaki Araki, Dexter Senft, David Smith and Ewart Shaw.
159 Peter Hilgers 72 Germany
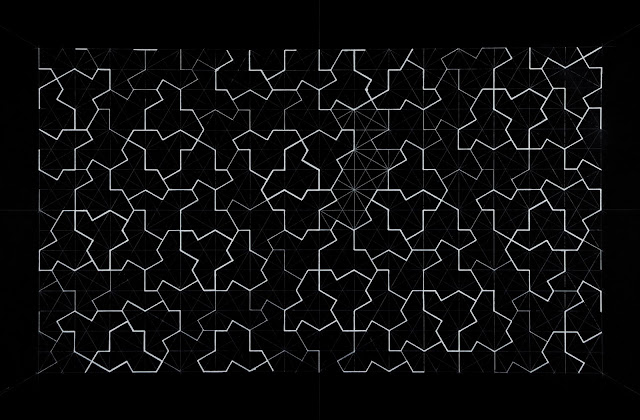
The image shows a look onto the infinite plane of the spectre tiling. On tile is selected, the initial patch P 0, and colored dark grey. It has 5 adjacent tiles which are called the first coordination shell P 1. In the n-th coordination shell P n are all tiles adjacent to P n-1 that are not in P n-2. The even coordination shells P 2 to P 16 are lowered.
Mathematical topic: The growth form, also known as corona limit, of a tiling may informally be defined as
lim Pn / n for n → ∞, if it exists. It is known that all periodic tilings do have a growth form [1]. Also many convex non-periodic tilings have a corona limit [2]. Recently it has been proved that the hat tiling has a growth form which is a regular hexagon [3]. It is supposed that this is true also for the spectre tiling. In both cases it is conjectured that the area of the hexagon is 2*Sqrt(3)*area of one tile.
The work was created with Mathematica and Blender.
[1] S. Akiyama, J. Caalim, K. Imai, H. Kaneko. “Corona Limits of Tilings: Periodic Case.” Discrete and Computational Geometry, vol. 61, no. 3, 2019, pp. 626-652
[2] D. Demski, P. Hilgers, and A. Shutov. “Growth forms of grid tilings.” Acta Crystallographica A,
vol. 78, no. 4, 2022, pp. 309-318
[3] A. Shutov. Personal communication
158 Emily Nevinson 26 UK
As a maths PhD student and someone who is into fibre arts, I thought it would be fun to make a quilted object using the hat tile instead of another typical quilt piecing shape! So I made a hat-themed quilted case for my e-ink tablet.
The patchwork fabric is made from hat tiles and pieced together using the English paper piecing method, which took 3 months to do alongside finishing my PhD work and starting to write my thesis. I then quilted this fabric to some wadding and made the tablet case (this step took significantly less time).
The first image is of the finished case, and the second has pictures of the front and back of the case and a picture of the fabric just after finishing the piecing.
157 Anna Alberska 31 Poland
'Mad Tea Party' (2023)
paper collage, 420x297 mm
I regret that we didn't know hat monotile yet in 2012, when I was designing my diploma thesis inspired by Alice's Adventures in Wonderland. The pattern this tile created would be a perfect complement to a paper costume for the Mad Hatter or a set design for a party he was attending. That's why now, after many years, I created a mosaic/collage of several hundred hand-cut paper hat tiles inspired by A Mad Tea Party.
156 Tyswan Slater 54 Australia
Windy Aperiodic Polykite (Mixed Media: ink on paper, digital).
155 Andrés Vindas Meléndez 30 USA
This is a planter that I made from styrofoam and duct tape. It is painted with gold metallic spray paint. The planter consists of live succulents which I grew with my mother in her home garden (she is pictured in the second photograph). She gave me permission to use the succulents for this project and I want to thank and credit her contribution.
154 Evan Brock 31 Canada
These ravioli bring together tessellation and fresh handmade pasta, for a more geometric dining experience.
Two wood molds were created to fill and shape the pasta, and 4 colors of dough was used to accentuate the tiling pattern. The mirrored tiles are made in the green (spinach) dough, while the yellow (turmeric), orange (carrot), and red (beet) doughs are all the standard version of the hat tile.
153 Verity Langley 16 UK
My entry is a Hat tile light box/mood lamp. It displays the hat tile design on your wall in many different relaxing colours (which it fades between). The hat tile lamp shade is made of black matt acrylic. I manufactured it by creating a TechSoft Design V3 design file, which consisted of each side of the shade's designs. Each side has finger joints on the long edges, so that they were able to be stuck together with glue to create the cuboid shape.
152 Stefan Hartmann 51 Germany
The big Einstein is composed of small Einsteins. Thus, the object defines the parquetry property and self-similarity. The small Einsteins were baked with shortcrust pastry and then decorated with colored marzipan and glued with icing - they are edible! For coloring, the four-color theorem was followed. Both of my sons helped me with baking and coloring and learned a lot of math as a result!
151 Ann Schwartz 74 USA
UPSIDE DOWN HAT FLEXAGON: Turn the now-famous hat upside down and it becomes a shirt!
A flexagon is a hinged polygon that displays a different appearance after it is manipulated in a particular way called "flexing." In his instructive book, "Flexagons Inside Out," author Les Pook shows a flexagon he calls "a compound ring of six hexagons." Seeing that his pyramidal honeycomb might be an excellent way to explore the hat's dynamics, I proceeded to make the flexagon and chose the "shirt" orientation.
The surfaces of a flexagon are called "faces:" this one has four. My first step was to put two interlocking shirts on each, making sure every face showed showed a different arrangement of tiles from the others. Then came the exciting part: flexing and seeing what happened. Would there always be at least one new interlocking shirt? Would there ever be two?
I discovered that three faces produced one new interlocking shirt after flexing. One face, the one that had its shirts neatly stacked on top of each other–the bottom of one shirt fitting snugly into the neckline of the other–produced TWO new shirts after flexing. A solid green shirt on top of a solid blue one turned into a yellow shirt on top of a blue shirt with orange sleeves–both stacked like the previous shirts.
The images attached show a more typical example. The first shows a pink shirt with a peace button. Upside down and adjacent to it is a purple shirt with gold buttons and the letter "R." The R indicates that the shirt is a reflection of the original tile. The second image shows what happens after flexing: a sailor shirt that is also a reflection.
I hope you enjoyed this "Einstein Mad Hat"–I certainly enjoyed making it–and thank you for your consideration. Thanks also to MOMATH and the United Kingdom Mathematics Trust, plus XTX Markets and all the other sponsors for their participation.
150 Matthew Thomas 35 UK
The key property of the einstein tiling is that it is aperiodic. This aperiodicity can be difficult to see and believe. Here the tiling is coloured to illustrate the aperiodicity. One surface of the tile is coloured red, the other grey. The brightness of the colour reflects the rotation of the tile. While not a proof, this presentation can help to visualise the aperiodicity.
149 Shiying Dong 41 USA
In this piece constructed with paper, the Tile(1, 1) is made chiral by having a pyramid on top of it. The apex of the pyramid is uniquely chosen so that it is equal distanced to five of the base vertices, which form ⅚ of a hexagon, and all the angles around the apex add up to 360 degrees, which allows the pyramid to be made without any gluing along the side edges. In this supertile layout, the Mystic tiles are highlighted with golden colors in the background of glimmering midnight blue.
148 James Bambrook 12 UK
Some people need maths to be fun in order to learn. That's why I've created 'Mad Hatterz' board game. People can learn about tessellation using the Einstein, one tile, hat design and have fun at the same time. It is a simple game for all ages and requires you to place tiles, joining on to each other to get the biggest score when you count how many sides have been joined. The game works every time and with any number of players (equipment supplies is for 1-4 people). Every game is different as players use logic and strategy to force each other into low scoring rounds. I love playing this game and in the future, extension packs could be added making it even more fun.
147 Fergus Sandys-McCormack 18 UK
I have created a puzzle using the Spectre tile as the shape of the pieces to showcase its tessellating properties. For the puzzle image, I created a Pop Art version of the famous picture of Einstein sticking out his tongue, to fit with the theme of the competition.
146 Joana Anjos 16 UK
A 67 piece puzzle, with the objective being to fit all copies of the hat tile together, within the set shape.
145 Denise Tiemi Miura 17 Brazil
My object is a wooden jigsaw puzzle. I wanted to play with the tiling concept and create a piece that could be aesthetically pleasing, fun to interact with and present a challenge. Because of the frame, you can only place the pieces in a certain way, but since they all have the same format but different heights and colors, you can create an infinity of patterns even though you're following the placement. It's made of four types of wood: Paricá, Tipuana and two different types of MDF. To create different colors and textures, some of them were painted, varnished and waxed.
144 Marta Czyrkiewicz 35 Poland
Bunny Tessellation:) Einstein shape, but in the form of a bunny.
143 Rosemarie Gallinari 62 USA
A monogrammed handkerchief
142 Emmaline Terlep 35 USA
This is my in-progress Einstein tile quilt. Due to the nature of the shape, it must be entirely hand-stitched using a quilting technique called English Paper Piecing. Each completed "hat" is 7 inches across the "brim." Ultimately, this will be a king-sized quilt of over 450 tiles in 7 colors. I currently have 6 different fabric colors, which have been arranged directionally in the layout photo. I have yet to select the color for my "mirror" tiles, but I'd like it to be something that stands out, maybe a garish green or metallic.
When a quilt is nearly finished, all the layers of fabric are held together with all-over stitching called quilting. For this quilt, I plan to overlay the whole thing with a triangular grid, using the tile edges as the guide. This should serve as a way to accentuate the mathematical nature of the patchwork without detracting from the tiles themselves.
141 Micha Kalle 21 Netherlands
Veneered artwork of the hat monotile pattern, with 4 different wood types. Meant to be hung like a painting.
140 Aimen Siddiqui 12 UK
139 Zoey Patterson 19 USA
Collage (with some paint) made primarily out of old calendars. Not created for this contest—I was just making a collage and decided to use the hat.
138 Astor Santos Neto 22 Brazil
I'm Astor Dilem dos Santos Neto, and I am an undergraduate student in Mathematics Education at the Federal University of Espírito Santo (UFES). I have a paper on planar tessellations with a focus on Penrose tiles, and I am currently studying the Hat.
My project aims to have an educational impact on elementary and high school students in Brazil. This is in line with the National Common Core Curriculum (BNCC), which requires students to be able to solve plane tiling problems with generalizations of patterns they observe and to calculate the measures of internal angles while establishing relationships between external and internal angles of polygons related to mosaics and tessellations.
Students are introduced to the concept of tiles and the unique aspects that make the Hat so special. They are then invited to try assembling the tiling. It's easy to notice that the majority of them become intrigued and motivated to put the tiles together. Moreover, they quickly realize that it's necessary to reflect some pieces and that there is a 'pattern' of spacing between them. Due to the absence of a strict rule for tilling, students may assemble it in a way that doesn't allow them to fill the entire plane. Therefore, at times, they need to disassemble part of what they've done and reassemble it differently.
This project is a work in progress but it's already a successful one, both the students and their teachers were happy to be a part of it.
137 Ichiro Tanioka 67 Japan
For many years I have been making animal puzzles for exhibits in science museums. The purposes are for children to experience the fun of tessellations while playing. Since aperiodic puzzles are among the most popular, I decided to create this new puzzle based on the amazing discovery, Spectre.
I have included the following features in this puzzle to make it more enjoyable for children. Firstly, for the design, we chose a dinosaur popular with many children and adopted Yoshiaki Araki's cute stegosaurus tessellation work. The material used is soft EVA resin, which can be safely assembled and interlocked by children without the use of glue. As an option, safe ferrite magnets can be embedded in the eye holes for children to play this puzzle on a steel wall like Tessellation Station in MoMath.
Another fun way to play this puzzle is to arrange it so that the neighboring pieces are of different colors. Spectre requires four colors for such arrangements, and children can easily experience examples of the famous mathematical theorem, Four Color Theorem with at least eight pieces.
The puzzle will be on permanent display at the HAMAGIN SPACE SCIENCE CENTER in Yokohama city, where an opening exhibition will be held from 23 December 2023, allowing visitors to experience the 600-piece puzzle.
136 Chelsea Lee 17 UK
As a mathematics and food enthusiast, I have always wanted merge them together. Hence, the idea of creating a tessellated Swiss roll with the iconic Hat and Spectre tiles combines the classic charm of a Swiss roll with an artistic twist.
135 AJ Bartoletti 38 US
The Hat / Aperiodic Monotile!
Hand-drawn on drafting table using strictly ruler and compass construction methods (no computer aid).
Technical Pens, Archival White Ink, Black Card.
Size: A3 – 16.5” x 11.7”
134 Petra Viola 16 USA
No description
133 Agnieszka Iwaszkiewicz 50 Poland
A collage using a fragment of Stanisław Bińkowski's painting "Portrait of a Woman". The woman's clothes reflect the shape of the Einstein Hat, and its geometric form perfectly matches Mr. Bińkowski's painting.
132 David Bailey 64 UK
Entry 1. Dog Heads (Dog Bone). Watercolour and Ink. A3 Bockingford Watercolour Paper
A hat tiling featuring two distinct (albeit alike) dogs, suitably combined in an innovative composition in different ways.
It will be seen that of an ‘upright’ hat (being asymmetrical), both the left and right-hand sides will permit a slightly different dog head. Therefore an obvious (and exciting) possibility is combining both views in a single composition (of course, both heads will also tile singly) to give added interest. For this, use is made of the possibility of using both unreflected and reflected tiles for the dogs (hence the disparity in the distribution of the motifs (as there are naturally more unreflected tiles than reflected).
Further, in keeping with the dog theme, the composition is self-contained within a (stylised) dog bone outline, just as an amusing ‘novelty’ presentation, rather than the more usually seen square or rectangular formats. Finally, the composition is centred on a dog in its most natural position, ‘upright’, as against a dog head ‘any which way’, which would be incongruous (i.e. at an unlikely angle). Although admittedly a minor consideration, such matters give a final pleasing touch to the tiling overall.
Colouration
Two (complementary) colours (orange and blue, for maximum contrast) are used, given the two dogs and two types of tile (as above), to reinforce the point and not the use of more colours (as with four or six colours commonly seen, of which the subtlety would then be lost of a casual glance). Of course, there is a trade-off here in terms of distinction, as the tiles are not map-coloured, but on occasion, such concerns can be overridden in terms of the demands of the innovative composition, which I think this fully justifies.
Entry 2. Birds (Hats within a Hat). Watercolour and Ink. A2 Bockingford Watercolour Paper
A hat tiling of birds with raised wings, within a hat outline.
A standard single bird tiling of the hat. In terms of innovation, of which little is possible here, the birds are shown within a hat outline, to as it were ‘emphasise’ the tile shape, which is thus made clear at a glance. The composition is centred on a bird (in red) in its most natural position, flying ‘upright’, echoing the orientation of the greater tile outline, which gives a nice unifying touch to the composition.
Colouration
A six-colouring scheme is chosen. Given that the tile appears in six orientations (including its reflection), an obvious thought is to use the six colours of the colour wheel, namely red, orange, yellow, green, blue, and violet as a unifying concept. Of course, there is a trade-off here in terms of distinction, as adjacent motifs of the same orientation have the same colour, and so the normally ‘ideal’ map colouring aspect is lost (a four-colouring is also possible, showing distinct tiles, but at the loss of ‘same orientations’, including reflections, as here). However, on occasion, such concerns can be overridden in terms of the demands of the greater colouring scheme, which I think this fully justifies.
A portrait of Albert Einstein made from aperiodic monotiles.
130 Celil Selcuk 14 UK
I have designed a puzzle that uses the Einstein hat tile instead of the traditional square piece. The main body is made out of wood, and instead of squaring off the pieces that would have gone off the edge, I have glued on a white-cardboard border (made up of identical tiles) to maintain the usual aperiodic tiling of the pieces. The cardboard and picture were all graphically designed on a computer, printed off, and cut out.
129 Grace Dunster-Ashworth 17 UK
No description
127 Mia Fan-Chiang 14 UK
I illustrated characters I could imagine within the aperiodic monotile and then turned some of them into biscuits. I did this because I believe that math is often seen as just complex equations when it actually has some amazing and beautiful things, like the aperiodic monotile. As well as that, art is often seen as the opposite of maths, although they do interlink. I believe the aperiodic monotile is an example of maths and art interlinking. I chose to make a few of these characters as biscuits because, just like food is part of our daily lives, so is maths.
126 Robert Mayes 16 UK
My submission is a deconstructable 3D model. The hexagonal structures are removable and rearrangeable to make a variety of different patterns. I like the idea of using this shape to make a repeating pattern.
I designed the individual pieces on a computer and used a 3D printer to make the individual tiles and I then glued them into the hexagonal structures. I also designed and printed the blue backing plates that hold the model together. The plates are stuck to a sheet of plywood that I cut to fit.