Einstein Hat Awards (entries 125-98)
Thank you to our sponsors, XTX Markets, UK Maths Trust, National Museum of Mathematics (MoMath.org), Amplify, G-Research, Jane Street and Dexter and Deborah Senft.
Contributors - Geoff Smith, Simon Coyle, Samuel Monnier, Dianne Flatt, Cindy Lawrence, Chaim Goodman-Strauss, Guillermo Acevedo, Kit Reagan, Hayley Richardson, Philipp Legner, Craig Kaplan, Robert Fathauer, Yoshiaki Araki, Dexter Senft, David Smith and Ewart Shaw.
125 Jason Cooper 46 Canada
Every year my family gets together for Thanksgiving, and as part of that, we spend a day building mugs, bowls, or other pottery. This year, I thought I'd take a crack at incorporating the new monotile!
The mug is hand-built by my mother, and painted in underglaze by me in a pattern that begins as the new tiling depicted in four colours with no adjacent tiles taking the same colour. Because, to my knowledge, the new tiling can't be done on a cylinder, the pattern departs from that toward the handle.
I had a lot of fun creating this. Hope you enjoy it as well!
124 Laurel Golash 64 USA
Spectre tessellation pot holder.
123 Jose Paulo Santiago 38 Philippines
When the Einstein Tiles first came out at the beginning of this year, I have started working on this project. Every student in my class received a tile, they decorated it, put their name on it and we put them together on the wall. I have explained to them the idea of diversity and international mindedness. Like the Einstein shapes that are aperiodic, we are all unique but we all fit in this world. Moreover, I also emphasized on the idea that anyone can be Einstein. We all have potential to be an agent of change in this world.
122 Nüzhet Merve Ertufan Haridasan 38 Turkey
I'm a visual artist based in Istanbul and Delhi. Recently I have completed an interactive artwork with the use of the Spectre tile, titled "Blinded by the same fog". It is made of two parts: the tile pattern on the wall with a starter text, and copies of these word-tiles on a nearby table. Using metal base and magnets, the audience is invited to play with those words, making their own combinations.
The size of the work can differ according to the size of the wall and the exhibition space. And, it can also be produced in any language, including English. In its recent rendition, the project was produced in Turkish in Türkiye, as can be seen in the pictures.
“Blinded by the same fog” was thoroughly inspired by the specific qualities of the newly developed einstein tile: a single geometric shape that can be tiled into infinity aperiodically. Which renders a single tile’s location incalculable in space, or in other words, it is impossible to predict in which orientation the tessellating tiles may proceed, leading to fresh clusters.
The project starts off with what I call the "starter text". An interconnected collection of coincidences that would (by suggestion) extend into infinity continuing outside the walls. Copies of these text tiles are then set on a nearby table, from which the audience can pick. Each exhibition starts with a new starter text, -a new set of coincidences-, and continues to develop until the whole wall is covered with text, rendering it unreadable. For MoMath, a version can be made where we ask beforehand for entries of interesting coincidences via open call on social media.
Using a text on coincidences overlaid on these tiles, “Blinded by the same fog” plays between the formal unpredictability in the world of geometry and the lived experience of chance and contingency.
121 Ellie Baker 69 USA
Ellie Baker
Title: “Aperiodic Flock”
digital print on silk fabric
36 by 36 inches
My process began with the creation of a Spectre tile outline based on the papers by David Smith, Joseph Samuel Myers, Craig S. Kaplan, and Chaim Goodman-Strauss. Using Adobe Illustrator, I constructed the tile as a vector graphic and carefully adjusted the curves to make it look to me more like a bird in flight. I drew the bird’s inner features by hand in pencil on paper and then took a photo of my drawing, which I aligned and combined with the vector graphic to create a finished tile that could be copied, pasted, and rotated within Illustrator. Using Craig Kaplan’s interactive application for producing patches of hat tiles, I made a large enough patch of hats whose tiling relationships I could follow to tile my birds within Illustrator. I thus created a patch (or flock!) of birds that would fit nicely on a 3 foot by 3 foot canvas and had the resulting image printed on silk fabric.
120 Ali Abbas 12 UK
Here is my entry for the Einstein Mad Hat awards.
I have fused my favourite sport (squash) and this mathematical concept into an experiment to see whether or not if I applied the Einstein aperiodic tiles onto a squash ball you would be able to make it perfectly without overlapping, following the usual form. This was answered as no, as when I got to tile number 8 overlapping occurred over number two– I put number one in the middle and spiralled from number two from the flat sheet I used for the template. I also put a picture of Einstein and an example his hat in the picture, as well as showing what the pattern would be like if it were flat.
119 Mosopefoluwa Mafe 16 UK
No description.
118 Blake Mason 11 UK
I made some tiffin for the competition. The tiffin is made using a family recipe with ingredients including butter, sugar, golden syrup, cocoa powder, biscuits, sultanas and lots and lots of chocolate!
My Mum makes tiffin for events in our village and it’s always a big hit, so I thought I’d make it for this competition and add the tessellation in white chocolate on the top to celebrate the design. I really enjoyed making it and being involved in the competition.
117 Arjan Nandhra 13 UK
My creation is a 72 panel football composed of 60 Einstein hats and 12 pentagons.
Five of the hats overpower the pentagon to create a panel. Twelve of these form a spherical shape to create a ball.
The ball is made of paper and card and hot glue filled with recycled paper from the project to help keep it's shape.
116 Preston McMurray 15 UK
This is my design of a Pokémon based on the einstein tile: Dreistein (so called because it is made if three stones).
115 Rehan Basu 11 UK
I've made a colourful collage featuring the "hat" tiles made from recycled paper from magazines, brochures and board games. Each tile has been hand-cut to create a kaleidoscopic effect. The hand-painted gold tiles represent the flipped ones. The collage is made using 60+ tiles. The nature of the aperiodic monotile means this can be expanded further in any direction. This concept can be explored further to create simple yet unique art pieces and mementoes.
114 Jolien Franke 31 Netherlands
It's a shirt. Q.E.D.
113 Issy Currie 16 UK
I have used the aperiodic monotiles to create a chessboard. As there are no longer ranks and files on the board, I have come up with new rules for how the pieces have to move. These are based on which side of the piece's current tile it has to cross over to get to the new tile. For example, the pawn can only cross over a side on its current tile that is coloured blue to get onto a new tile. The full rules for all of the pieces are as below:
King: Can cross over any of the edges of the tile, and can move a maximum of one tile in each turn.
Queen: Can cross over any edge and can move a maximum of five tiles at a time.
Rook: Must cross over a blue or green side on its current tile, a maximum of three tiles at a time
Bishop: Must cross over a red side, maximum of three tiles.
Knight: Must move exactly three tiles in a turn – one move must cross over a red side, one over a blue side, and one over a green side, in any order, and it can jump over other pieces like in regular chess.
Pawn: on the first move it can move one tile and cross over any side, but cannot take another piece on the first go. On all other moves, it must cross over a blue side, a maximum of one tile at a time.
None of the pieces are allowed to pass over a tile more than once in a single turn.
112 Adhila Salisu 16 UK
I tie-dyed a shirt in spiral patterns in red and purple with the Einstein hat tile shape painted on the shirt where a small symbol would be. This is a play on pattern on pattern. A shape with the potential to make a non repeating pattern on a repeating spiral pattern. I took 3 days to dye and dry the shirt.
111 Christian Walther 42 Switzerland
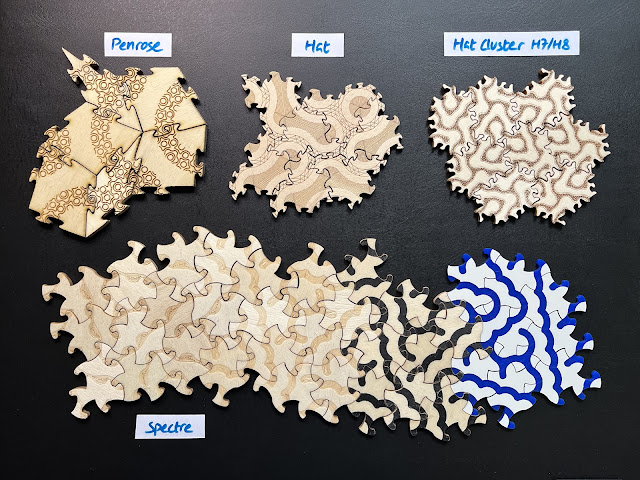
This jigsaw puzzle makes hands-on exploration of the Spectre aperiodic tiling fun!
Physical tiles are a great way to playfully explore an aperiodic tiling and form an intuition about its properties. In their most basic form though, with straight edges, the tiles have a distinct disadvantage: The arrangement that you spent so much time carefully aligning on your desk is easily destroyed by an accidental brush of the hand that scatters it into pieces.
My way to solve that is to add jigsaw-puzzle-style blanks and tabs to the edges of the tiles, so that they hold together, and an assembled patch can be shaken around without falling apart. I first did that with the Penrose tiling a few years ago, where the tabs additionally form a natural encoding of the matching rules that are usually indicated with markings on the tiles – the jigsaw tiles simply don’t fit together in the wrong way.
The Penrose puzzle turned out quite fun to play with, so of course I had to try the same thing when I heard of the Hat and Spectre aperiodic monotiles. The Hat prototypes turned out to be a disappointment: It was much too difficult to assemble larger patches without quickly ending up in dead ends where no more piece would fit. This is probably due to the specific arrangement of reflected tiles in the aperiodic tiling, which is hard to get right intuitively.
The Spectre puzzle piece that is presented here, on the other hand, made me quite happy. The shape is simple: only a curved edge, with no complicated squigglies, similarly to what is proposed in the paper to exclude reflections, is sufficient on the basic Spectre shape to make the pieces interlock in almost all of the ways they fit together. Assembling the puzzle is challenging in just the right way – more difficult than the Penrose tiling, but less so than the Hat tiling. You sometimes end up in dead ends and need to backtrack, but over time you develop an intuition of which arrangements work and which lead to trouble.
Additionally, it is also possible to assemble the pieces to beautiful non-aperiodic patterns with various symmetries if you deliberately allow holes in the tiling.
The decorative Truchet-like pattern that is printed on the tiles always lines up, no matter which way the tiles are connected. Coming up with a nice pattern with that property required a few iterations of prototypes. In the aperiodic tiling, the lines form a peculiar characteristic pattern with triangular features. It looks like they connect to a single, infinite, simply-connected shape, although I have not proved that. This property also provides help when assembling the puzzle: If you get loops or disconnected pieces in the pattern, that is an indication that you will later run into trouble when trying to extend the patch further.
The puzzle pieces are laser-cut from plywood or acrylic. The markings were initially laser-engraved on the wood as well, but I found that to provide too little contrast to make the pattern clearly visible, so I later settled on spray-painting with a laser-cut stencil. Laser-engraving a bi-color acrylic material was tried as well, but did not yield satisfactory results.
Links:
More photos and development updates: https://mstdn.social/@isziaui
Drawings and laser-cut files: https://github.com/cwalther/monotile-puzzle
Me presenting the tiles in a YouTube show (25 min): https://youtu.be/Iw-KhkTb5wY?t=163
It should be noted that other people have independently come up with the same idea: https://n-e-r-v-o-u-s.com/blog/?p=9333 . I was not aware of that project during my development and only learned of it recently.
110 Nancy Clarke 10 UK
I wanted to make a very large, impactful piece that would catch someone’s eye. I used two bold shades, that were completely different so the hat would pop out. I wanted to make a bigger piece, so using eight small Einstein tiles (altogether), I made two huge Einstein tiles: one black and one white. Then, I went around the outlines of the small Einstein tiles within each big piece with masking tape. Afterwards I intersected them and stuck them to a plain black hair band.
108 Damiano Incisa di Camerana 16 UK
No description.
107 Amal Salisu 16 UK
I created a key chain made out of polymer clay in the shape of the Einstein hat tile. I constructed the shape and used it as a stencil for the clay, and painted it blue.
106 Elliot Brackley 16 UK
I have created a model of the theory of relativity in a 3D digital form, using the Einstein hat shape to create a pattern that covers the star, the planet and everything around it. This includes the dip created by the bending of space time.
105 Saara Ali Afzal 11 UK
My name is Saara.
This is my entry for the Einstein National Competition. By using a diagram of my tile I have created a tile collage. I have used different materials such as felt, craft foam, and card in a variety of colours , textures and patterns.
104 Oskar Eriksson-Lee 24 UK
In tribute to the discovery of the aperiodic monotile, I present a deltahedral triakis icosahedron - featuring a wrap-around Hat tiling and visually inspired by the Rosette Nebula.
From the onset of this project I aimed to explore the intersection of three of my passions: geometry, art and space. When I realized that the Hat could be tiled with three-fold rotational symmetry I became resolved on creating a deltahedron by using a Hat tiling-based net.
Using a tetrahedron as my starting point for experimentation I eventually landed on a specific periodic tiling (combining Hats and alternating kites) that allows for the construction of the nets for all deltahedra. Of all the various deltahedra this tiling could now be assembled from, the one that stood out to me most was the non-convex triakis icosahedron with its complex star-like form.
Alternating in colour upon the triakis icosahedron; the Hat tiles with their tendril-like pattern evoked a similarity to that of the spiralling wisps of cosmic dust and ionized gas characteristic of interstellar nebulae. From many angles it struck me as sharing a particular likeness to the Rosette Nebula, located within the same region of space as the Monoceros constellation. To that end I decided on a colour scheme of red and two shades of blue to represent, respectively, the H-alpha and O III emissions of the Rosette Nebula.
*Alongside the primary picture, I also include an animated .gif to better showcase the three-dimensionality of the deltahedral triakis icosahedron.
103 Ursa Ogilvie Thompson 17 UK
For my design I wanted to make something fun that took advantage of the mathematical shape of the tile. I decided to use the fact that the tile is made up of 8 kite shapes to make one big, literal kite in the shape of the hat tile, made up of 8 smaller, also literal kites, in the shape of a traditional kite, which can either be flown separately or be put together and flown as a big ‘hat tile kite’.
I first found the ratio of the lengths required of the vertical and horizontal lengths of each kite shape and then scaled them to my desired lengths which I decided to be 57.7cm for the vertical and 50cm for the horizontal. I used these measurements to make 8 kites from brown paper. I then cut out a smaller scale hat tile out of cardboard (with kite dimensions of 3.5cm(horizontal)x 4.04cm(vertical)). I worked out how many smaller hat tiles were required to cover the area of my 8 kites and then used the cardboard tile as a template to make the required number of hat tiles out of paper which I then painted and used to cover the surface of each large kite in the aperiodic pattern. I then glued sticks across the horizontal and vertical lengths as well as the sides of each kite to make them more stable and be able to keep their shape. Once all of the kites were finished I glued on square shapes of the loop side of Velcro on the back, two pieces on each side, one on each corner. I then cut lengths of Velcro hooks two times the length of the squares which allowed me to connect the kites in any order I wanted them in. Once they were connected I finished the kite by tying a piece of string onto each of the kites which made it/them able to fly.
The big kite can be flown on its own (as seen in the second picture (it wasn’t particularly windy so it didn’t fly as much as it could)) and it can also be taken apart and rearranged into any other variation of the hat tile the person wishes or each smaller kite can be flown separately therefore being a fun design for one or multiple people.
102 Garnet Frost 70 UK
24 ft External Handcrafted Tiled Frieze
By Garnet Frost and The Alhambra Tiling Project
Our submission to the contest is our incredible 24ft tiled frieze. Comprising over 1,500 ceramic tiled pieces. This frieze will soon take pride of place along the upper frontage of our London Ceramic Workshop (see photo).
Every single tile has been lovingly handmade and shaped, primarily using handmade tools and equipment. A group of dedicated community volunteers have put in hundreds of hours of work, led by me, Garnet Frost, and advised by expert ceramist, Matthew Taylor.
The installation will occur in the coming weeks, once we are satisfied that waterproofing and safety issues have been addressed.
For various reasons, including illness, this project has proven to be very challenging. Strangely enough, it has also been an energising and life-affirming adventure—one that seemed to come from nowhere and yet has blossomed into a remarkable and breathtaking accomplishment.
Special Thanks
To David Smith for his inspirational enthusiasm and encouragement. As we worked tirelessly, we were constantly aware of the beauty and simplicity of the Hat Tile.
Footnotes:
The Alhambra Tiling Project is a not-for-profit social enterprise with an educational purpose. Most of the tiles on the end wall in the main photo were actually crafted by primary school children. I have also collaborated with patients at The Royal Bethlem Hospital, as well as users of the food bank 'Living Well' in Bromley, and other disadvantaged groups.
Should we secure grant funding, e.g., from The Arts Council or the G.L.A., we aim to undertake more large-scale work with the Hat and/or the Spectre, engaging many more participants. Regardless of the outcome of this competition, our involvement will significantly bolster our credentials. Final thanks to everyone who has made this happen.
We have been keeping a photo log of our progress while making the hat Frieze.
https://instagram.com/aperiodicadventures
101 Youngjoo Kim 14 Korea
This is the mixture of the buildings; the Louvre Pyramid, the Shard and the Flatrion building.
The bottom part has the same part as the side with the building of the Shard. The three-sided parts are related to the side shape of the Livre Pyramid. Lastly, the side part of the triangular shape has the same part as the top of the building Flatrion.
The three colours; yellow blue and black each represent the colour of the buildings I have used. Blue is the colour of the Shard. As it has the same colour as the exterior part of the building. Hello is used to represent the Louvre Pyramid. When the sun penetrates the pyramid, it changes to a certain colour and the most strong colour of the yellow. Black represents the colour of the building Flatrion. This building is mostly covered with grey and this grey becomes black when it is strongly mixed.
100 Seth Cooper 41 USA
In this project I wanted to focus on the edges between the tiles rather than the tiles themselves. I converted the tile locations into a network of edges, and then color those edges based on an underlying image. In this case, the underlying image is of the hat tiles at a larger scale, giving a "hat-of-hats" kind of look to it. The smaller hat edges give an interesting, noisy texture to the image, and theoretically any underlying image could be used. In the second animated image, the hat edge network is explored somewhat randomly, slowly revealing the underlying image. (The animation quality is somewhat low in the submission due to the gif format.)
This project was based on the tools available here:
https://cs.uwaterloo.ca/~csk/hat/
https://github.com/isohedral/hatviz
99 Sresht Seethanaboina 11 UK
I made a puzzle out of the Einstein Hat Tiles by printing, cutting and decorating them. Then I had some fun joining them and trying to find out a way that they don't make any holes although they ended up with many holes. It was quite tricky but a lot of fun as it was challenging and exercised my brain! The shape was irregular and had an odd number of sides.
98 Katie Woolard 13 UK
Katie has handcrafted and painted a cat wearing the Einstein tile.